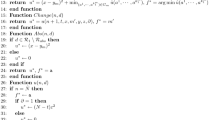Skip to main content
Springer Nature Link
Log in

Tracking error with minimum guarantee constraints

  • Conference paper
Mathematical and Statistical Methods for Actuarial Sciences and Finance

  • 2490 Accesses

Abstract

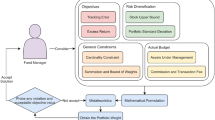
In recent years the popularity of indexing has greatly increased in financial markets and many different families of products have been introduced. Often these products also have a minimum guarantee in the form of a minimum rate of return at specified dates or a minimum level of wealth at the end of the horizon. Periods of declining stock market returns together with low interest rate levels on Treasury bonds make it more difficult to meet these liabilities. We formulate a dynamic asset allocation problem which takes into account the conflicting objectives of a minimum guaranteed return and of an upside capture of the risky asset returns. To combine these goals we formulate a double tracking error problem using asymmetric tracking error measures in the multistage stochastic programming framework.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
USD 29.95
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Alexander, G.J., Baptista, A.M.: Portfolio selection with a drawdown constraint. J. Banking Finan. 30, 3171–3189 (2006)

    Article  Google Scholar 

  2. Barro, D., Canestrelli, E.: Tracking error: a multistage portfolio model. Ann. Oper. Res. 165(1), 44–66(2009)

    Article  MathSciNet  Google Scholar 

  3. Basak, S.: A general equilibrium of portfolio insurance. Rev. Finan. Stud. 8, 1059–1090 (1995)

    Article  Google Scholar 

  4. Basak, S., Shapiro, A., Tepla, L.: Risk management with benchmarking. Working paper series asset management SC-AM-03-16, Salomon Center for the Study of Financial Institutions, NYU (2003)

    Google Scholar 

  5. Boyle, P., Tian, W.: Optimal portfolio with constraints. Math. Finan. 17, 319–343 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  6. Brennan, M., Solanki, R.: Optimal portfolio insurance. J. Finan. Quant. Anal. 16, 279–300 (1981)

    Article  Google Scholar 

  7. Browne, S.: Beating a moving target: optimal portfolio strategies for outperforming a stochastic benchmark. Finan. Stochastics 3, 255–271 (1999)

    MathSciNet  Google Scholar 

  8. Consiglio, A., Cocco, F., Zenios, S.A.: The Prometeia model for managing insurance policies with guarantees. In: Zenios, S.A. and Ziemba, W.T. (Eds.) Handbook of Asset and Liability Management, vol. 2, pp. 663–705. North-Holland (2007)

    Google Scholar 

  9. Consiglio, A., Saunders, D., Zenios, S.A.: Asset and liability management for insurance products with minimum guarantees: The UK case. J. Banking Finan. 30, 645–667 (2006)

    Article  Google Scholar 

  10. Deelstra, G., Grasselli, M., Koehl, P.-F.: Optimal investment strategies in the presence of a minimum guarantee. Ins. Math. Econ. 33, 189–207 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  11. Deelstra, G., Grasselli, M., Koehl, P.-F.: Optimal design of the guarantee for defined contribution funds. J. Econ. Dyn. Control 28, 2239–2260 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  12. Dembo, R., Rosen, D.: The practice of portfolio replication, a practical overview of forward and inverse probems. Ann. Oper. Res. 85, 267–284 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  13. Dempster, M.H.A., Thompson, G.W.P.: Dynamic portfolio replication usign stochastic programming. In: Dempster, M.H.A. (ed.) Risk Management: Value at Risk and Beyond, pp. 100–128. Cambridge Univeristy Press (2001)

    Google Scholar 

  14. Dempster, M.A.H., Germano, M., Medova, E.A., Rietbergen, M.I., Sandrini, F., and Scrowston, M.: Designing minimum guaranteed return funds. Research Papers in Management Studies, University of Cambridge, WP 17/2004 (2004)

    Google Scholar 

  15. El Karoui, N., Jeanblanc, M., Lacoste, V.: Optimal portfolio management with American capital guarantee. J. Econ. Dyn. Control 29, 449–468 (2005)

    Article  MATH  Google Scholar 

  16. Franks, E.C.: Targeting excess-of-benchmark returns. J. Portfolio Man. 18, 6–12 (1992)

    Article  Google Scholar 

  17. Gaivoronski, A., Krylov, S., van der Vijst, N.: Optimal portfolio selection and dynamic benchmark tracking. Eur. J. Oper. Res. 163, 115–131 (2005)

    Article  MATH  Google Scholar 

  18. Grossman, S.J., Zhou, Z.: Equilibrium analysis of portfolio insurance. J. Finan. 51, 1379–1403 (1996)

    Article  Google Scholar 

  19. Halpern, P., Kirzner, E.: Quality standards for index products. Working paper. Rotman School of Management, University of Toronto (2001)

    Google Scholar 

  20. Hochreiter, R., Pflug, G., Paulsen, V.: Design and management of Unit-linked Life insurance Contracts with guarantees. In: Zenios, S.A. and Ziemba W.T. (eds.) Handbook of asset and liability management vol. 2, pp. 627–662. North-Holland (2007)

    Google Scholar 

  21. Jensen, B.A., Sorensen, C.: Paying for minimal interest rate guarantees: Who should compensate whom? Eur. Finan. Man. 7, 183–211 (2001)

    Article  Google Scholar 

  22. Konno, H., Yamazaki, H.: Mean absolute deviation portfolio optimization model and its applications to Tokyo stock market. Man. Sci. 37, 519–531 (1991)

    Google Scholar 

  23. Lintner, J.: The valuation of risky assets and the selection of risky investments in stock portfolios and capital budget. Rev. Econ. Stat. 47, 13–37 (1965)

    Article  Google Scholar 

  24. Michalowski, W., Ogryczak, W.: Extending the MAD portfolio optimization model to incorporate downside risk aversion. Naval Res. Logis. 48, 185–200 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  25. Mossin, J.: Equilibrium in a Capital Asset Market. Econ. 34, 768–783 (1966)

    Google Scholar 

  26. Muermann, A., Mitchell, O.S., Volkman, J.M.: Regret, portfolio choice, and guarantees in defined contribution schemes. Insurance: Math. Econ. 39, 219–229 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  27. Rudolf, M., Wolter, H.-J., Zimmermann, H.: A linear model for tracking error minimization. J. Banking Finan. 23, 85–103 (1999)

    Article  Google Scholar 

  28. Sharpe, W.F.: Capital asset prices: a theory of market equilibrium under conditions of risk. J. Finan. 19(3), 425–442 (1964)

    Article  MathSciNet  Google Scholar 

  29. Sorensen, C.: Dynamic asset allocation and fixed income management. J. Finan. Quant. Anal. 34, 513–531(1999)

    Article  Google Scholar 

  30. Zenios, S.A., Ziemba, W.T. (eds.): Handbook of Asset and Liability Management, vol. 2. North-Holland (2007)

    Google Scholar 

  31. Ziemba, W.T.: The Russel-Yasuda Kasai, InnoALM and related models for pensions, Insurance companies and high net worth individuals. In: Zenios, S.A., Ziemba, W.T. (eds.) Handbook of Asset and Liability Management, vol. 2, pp. 861–962. North-Holland (2007)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Applied Mathematics, University Ca’ Foscari Venice, Venice, Italy

    Diana Barro & Elio Canestrelli

Authors
  1. Diana Barro
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Elio Canestrelli
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. Department of Applied Mathematics, University Ca’ Foscari Venice, Venice, Italy

    Marco Corazza

  2. Department of Statistics, University Ca’ Foscari Venice, Venice, Italy

    Claudio Pizzi

Rights and permissions

Reprints and permissions

Copyright information

© 2010 Springer-Verlag Italia

About this paper

Cite this paper

Barro, D., Canestrelli, E. (2010). Tracking error with minimum guarantee constraints. In: Corazza, M., Pizzi, C. (eds) Mathematical and Statistical Methods for Actuarial Sciences and Finance. Springer, Milano. https://doi.org/10.1007/978-88-470-1481-7_2

Download citation

Publish with us

Policies and ethics