Bargaining Power and Value Sharing in Distribution Networks: A Cooperative Game Theory Approach

Abstract

This paper introduces a novel methodology for analyzing bargaining games on network markets, which are markets where transactions occur by means of distribution networks (e.g., gas, electric energy, water, etc.). The overall economic surplus obtained in the market is distributed among all network agents the on the basis of their bargaining power, which in turn depends on a variety of factors: position of each agent (e.g., a country) in the network, reliability in the cooperation scheme (e.g., geo-political stability), existence of market distortions and availability of outside options (e.g., alternative energy sources). The method we propose, which is illustrated here through an application to a fictitious network structure, is based on a two-stage process: first, a network optimization model is used to generate payoff values under different coalitions and network structures; second, cooperative game solutions are identified. Any change in the network structure entails both a variation in the overall welfare level and in the distribution of surplus among agents, as it affects their relative bargaining power. Therefore, expected costs and benefits, at the aggregate as well as at the individual level, can be compared to assess the economic viability of any investment in network infrastructure. A number of model variants and extensions are also considered: changing demand, exogenous instability factors, market distortions, externalities and outside options.

Appendix: Using the Nucleolus to allocate the cooperative surplus

The Shapley value is not the only equilibrium concept elaborated in cooperative game theory, and other allocation rules could be adopted in the analysis of cooperative networks. The nucleolus, for example, is another concept, appealing due to its relation to the “core” of a cooperative game (Schmeidler 1969). The core is the set of all possible allocations in which the sum of values assigned to the members of any coalition is at least equal (or greater) than the surplus obtainable by the coalition, acting autonomously. The core may be empty but, when it is not, it often includes many allocations, which limits its applicability. The nucleolus is that allocation which lies in the “lexicographical center” of an non-empty core (Maschler et al. 1979).

Although the nucleolus has a logic and appealing interpretation, its computation for large networks may turn out to be quite complicated and the interpretation not easy. For this reason, we preferred to illustrate the basic ideas in this paper using the Shapley value, which is simpler to compute and possesses a number of desirable properties (e.g., monotonicity and linearity). However, it is not too difficult to compute the corresponding nucleolus values for the simple network discussed in the paper. Here we present our findings, using the nucleolus instead of the Shapley value.

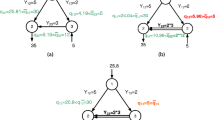
Table 7 corresponds to Table 1. With the nucleolus, demand nodes B, C and D gets relatively more value in the baseline. The effect of expanding capacity in the link B-D is qualitatively similar to the Shapley case, with two differences: (1) node A is harmed, (2) benefits for B and D are not symmetric. Indeed, symmetry is a special property of the Shapley value.

Table 7 Nucleolus value surplus distributions

Table 8 corresponds to Table 2, where the case of expanding demand in node C is considered. Again, effects are qualitatively similar, but now the benefits accrue primarily to node C, whereas D and E are completely unaffected by the change in market size.

Table 8 Nucleolus value surplus distributions

Table 9 corresponds to Table 3. Here we consider the existence of “exogenous instability” in node C. Of course, allocations change both in the baseline and after the relaxation of capacity constraints. The gains or losses for A, B and C are almost unaffected by the presence of instability, whereas gains for D and losses for E are amplified. Again, the impact of investment in capacity in the link B-D are not symmetric for nodes B and D.

Table 9 Nucleolus distributions with instability in C

Table 10 corresponds to Table 4. The existence of an outside option in market D strengthen the bargaining power of D and its value share, but much less than in the Shapley case. Actually, most of the gains now accrue to nodes B and C (slightly harmed under Shapley), whereas node E is unaffected (significantly harmed under Shapley).

Table 10 Nucleolus distributions with outside option in node D

Table 11 corresponds to Table 5, comparing the payoffs of the base case with the ones obtained when an import quota of 40 is introduced for node C. The import constraint reduces welfare for the agents A, B, and C, whereas D and E are unaffected. Contrary to Shapley, welfare losses are not symmetric, but accrue primarily to C.

Table 11 Nucleolus distributions with import quota in node C

Table 12 corresponds to Table 6. The incidence of exogenous factors is the same when the nucleolus replaces the Shapley value as an allocation rule.

Table 12 Nucleolus distributions with exogenous surplus factors